Home
Physics Simulation
Simcenter STAR-CCM+ can model a wide range of physics phenomena including fluid mechanics, solid mechanics, heat transfer, electromagnetism, and chemical reactions. Scenarios with multiple time scales can be solved within the same simulation.
Turbulence
Most fluid flows of engineering interest are characterized by irregularly fluctuating flow quantities.
Selecting a Turbulence Modeling Approach
Selecting the turbulence modeling approach is possible with or without auto-select.

Share

Link: copied

Breadcrumb: copied

Physics Simulation
Simcenter STAR-CCM+ can model a wide range of physics phenomena including fluid mechanics, solid mechanics, heat transfer, electromagnetism, and chemical reactions. Scenarios with multiple time scales can be solved within the same simulation.
- Defining Physics Workflow
  Simcenter STAR-CCM+ contains a wide range of physics models and methods for the simulation of single- and multi-phase fluid flow, heat transfer, turbulence, solid stress, dynamic fluid body interaction, aeroacoustics, and related phenomena. These physics models are all selected using a physics continuum.
- Setting Up Physics
  This section provides information on how to set up physics models in STAR-CCM+.
- Running Physics Simulations
  This part of the documentation describes preparation and procedures for running a Simcenter STAR-CCM+ simulation.
- Motion
  In general, motion can be defined as the change in position of a body with respect to a certain reference frame.
- Space
  The primary function of the Space models in Simcenter STAR-CCM+ is to provide methods for computing and accessing mesh metrics. Examples of mesh metrics include cell volume and centroid, face area and centroid, cell and face indexes, and skewness angle.
- Time
  The primary function of the time models in Simcenter STAR-CCM+ is to provide solvers that control the iteration and/or unsteady time-stepping.
- Mesh Motion and Adaption
  Many simulations that involve motion or geometry change require you to move or deform the mesh. Other simulations require localized mesh adaption in order to achieve an accurate solution.
- Materials
  Material models simulate substances, including various mixtures.
- Fluid Flow
  Many engineering design projects require you to predict the effect of flowing fluids on containing structures or immersed objects. While you can analyse simple scenarios with hand calculations, complex scenarios require you to apply numerical methods for accurate solutions.
- Viscous Flow
  Viscous Flow is a finite element approach for use with viscoelastic materials and other highly viscous non-Newtonian fluids, such as liquid plastics and rubber, dough and similar foodstuffs, molten glass, and mud. Viscoelastic materials resemble elastic materials, but also exhibit viscous effects, rebounding slowly from deformations.
- Passive Scalars
  Passive scalars are user-defined variables of arbitrary value, assigned to fluid phases or individual particles. They are passive because they do not affect the physical properties of the simulation. An intuitive way to think of passive scalars is as tracer dye in a fluid, but with numerical values instead of colors, and with no appreciable mass or volume.
- Heat Transfer
  Heat transfer is the study of energy in transit due to a temperature difference in a medium or between media. Heat transfer extends thermodynamic analysis through the study of the modes of energy transfer and through development of relations to calculate energy transfer rates.
- Species
  This chapter provides information about the chemical species models in Simcenter STAR-CCM+. A species model is activated whenever a multi-component liquid or multi-component gas is chosen from the Material model section of the Physics Model Selection dialog.
- Porous Media
  Simcenter STAR-CCM+ allows you to simulate the transport of a fluid or energy (e.g. heat, electrical charge) through porous materials using the concept that the action of the porous media can be represented using appropriate loss (or 'diffusion') coefficients.
- Adjoint
  The adjoint method is an efficient means to predict the influence of many design parameters and physical inputs on some engineering quantity of interest, that is, on the engineering objective of the simulation. In other words, it provides the sensitivity of the objective (output) with respect to the design variables (input).
- Fans and Blowers
  Simcenter STAR-CCM+ provides models for axial and radial fans where classical fan laws apply.
- Virtual Disk Model
  The virtual disk model is based upon the principle of representing propellers, turbines, rotors, fans, and so on, as an actuator disk. The actuator disk treatment is practical when you are concerned about the influence of the rotor/propeller behavior on the flow rather than knowing about the detailed interactions between the flow and the blades of the rotating device.
- Turbulence
  Most fluid flows of engineering interest are characterized by irregularly fluctuating flow quantities.
  - Selecting a Turbulence Modeling Approach
    Selecting the turbulence modeling approach is possible with or without auto-select.
  - Reynolds-Averaged Navier-Stokes (RANS) Turbulence Models
    RANS turbulence models provide closure relations for the Reynolds-Averaged Navier-Stokes equations, that govern the transport of the mean flow quantities.
  - Scale-Resolving Simulations
    In contrast to RANS models, scale-resolving simulations resolve the large scales of turbulence and model small-scale motions.
  - Wall Treatment
    Walls are a source of vorticity in most flow problems of practical importance. Therefore, an accurate prediction of flow and turbulence parameters across the wall boundary layer is essential.
- Transition
  The term transition refers to the phenomenon of laminar to turbulence transition in boundary layers. A transition model in combination with a turbulence model predicts the onset of transition in a turbulent boundary layer.
- Wall Distance
  Wall distance is a parameter that represents the distance from a cell centroid to the nearest wall face with a non-slip boundary condition. Various physical models require this parameter to account for near-wall effects.
- Radiation
  The Radiation Model is the gateway, or entry point to all of the radiation modeling capabilities of Simcenter STAR-CCM+. This section describes Simcenter STAR-CCM+’s radiation modeling.
- Aeroacoustics
  Aeroacoustics investigates the aerodynamic generation of sound.
- Reacting Flow
  Simcenter STAR-CCM+ provides a selection of models that you can use to simulate a wide range of reacting flow applications.
- Internal Combustion Engines
  In an internal combustion engine (ICE), for example a gasoline engine, the process of combustion takes place in a cylinder (or cylinders) within the engine. The working fluid is a fuel and oxidizer mixture (usually air), which reacts to form combustion products.
- Multiphase Flow
  Multiphase flow is a term which refers to the flow and interaction of several phases within the same system where distinct interfaces exist between the phases. Simcenter STAR-CCM+ considers flow options where phases coexist as: gas bubbles in liquid, liquid droplets in gas, and/or solid particles in gas or liquid, and/or (large scale) free surface flows.
- Dynamic Fluid Body Interaction
  Dynamic Fluid Body Interaction (DFBI) in Simcenter STAR-CCM+ allows you to simulate the motion of a 6-DOF body with the displacement and rotation resulting from the defined mechanical and multiphysics interaction (flow, DEM, solid stress, EMAG).
- Harmonic Balance
  Some unsteady flows have a regularly repeating flow pattern, that is, they are time-periodic. Consider the flow from a fan blade passing across the entrance to a duct. Measurements of the instantaneous flow just within the duct would show a regularly repeating pattern. If the flow disturbances are sufficiently large, and propagate to the end of the duct, measurements of the unsteady flow at any point within the duct show repeating patterns. Such time-periodic patterns can be expressed using Fourier series.
- Solid Stress
  Simcenter STAR-CCM+ allows you to model the response of a solid continuum to applied loads, including mechanical loads and thermal loads that result from changes in the solid temperature.
- Electromagnetism
  Simcenter STAR-CCM+ allows you to model engineering applications involving electromagnetic phenomena. Example applications are electric motors, electric switches, and transformers, which can be modeled based on the classical theory of Electromagnetism.
- Electrochemistry
  Electrochemistry is the study of chemical reactions which occur due to an imposed electrical charge, or a difference in electrical potential at a boundary between a conductor–such as a metal–and an electrolyte. Simcenter STAR-CCM+ provides models that allow you to simulate batteries, corrosion, etching, and other electrochemical reactions.
- Electric Circuits
  Electrical circuits are conducting loops of interconnected electrical components, such as batteries, power sources, resistors, and inductors.
- Plasma
  Plasma is a state of matter similar to a gas that is composed partially or completely of charged particles such as ions and electrons which are not bound to each other.
- Batteries
  You can simulate batteries in Simcenter STAR-CCM+ using battery cells and battery cycling procedures that are defined either directly in Simcenter STAR-CCM+, or in the external software package Simcenter Battery Design Studio.
- Casting
  Casting simulations are performed using transient multiphase simulations using the VOF model with solidification. Conjugate heat transfer is applied between the solidifying melt and the solid mold.
- Region Sources
  This section provides some guidelines on how to use region sources for some common problems.
- Cell Quality Remediation
  The Cell Quality Remediation model helps you get solutions on a poor-quality mesh. This model identifies poor-quality cells, using a set of predefined criteria, such as Skewness Angle exceeding a certain threshold. Once these cells and their neighbors have been marked, the computed gradients in these cells are modified in such a way as to improve the robustness of the solution.
- Guidelines for Applying Simcenter STAR-CCM+
  This part of the documentation provides guidelines on applying Simcenter STAR-CCM+ models to your applications.
- Common Solvers Reference
  In Simcenter STAR-CCM+, solvers compute the solution during the simulation run.

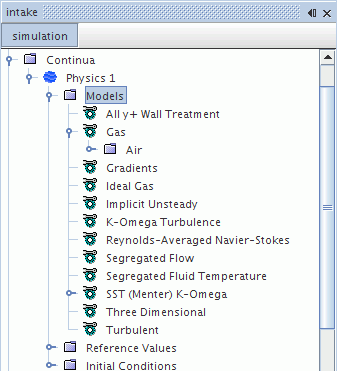
Selecting a Turbulence Modeling Approach

Selecting the turbulence modeling approach is possible with or without auto-select.

Using Auto-Select

This section describes how to select a turbulence modeling approach using automatic selection of recommended models.

This is the default mode of selection, and is indicated by the Auto-select recommended physics models checkbox being ticked.

The selection of the Turbulent radio button in the Viscous Regime group box will automatically select turbulence models that provide closure of the Reynolds-Averaged Navier-Stokes (RANS) equations as a turbulence modeling approach. These modeling choices will be displayed in the Reynolds-Averaged Turbulence group box as shown below, although the choice of Enabled Models might differ.

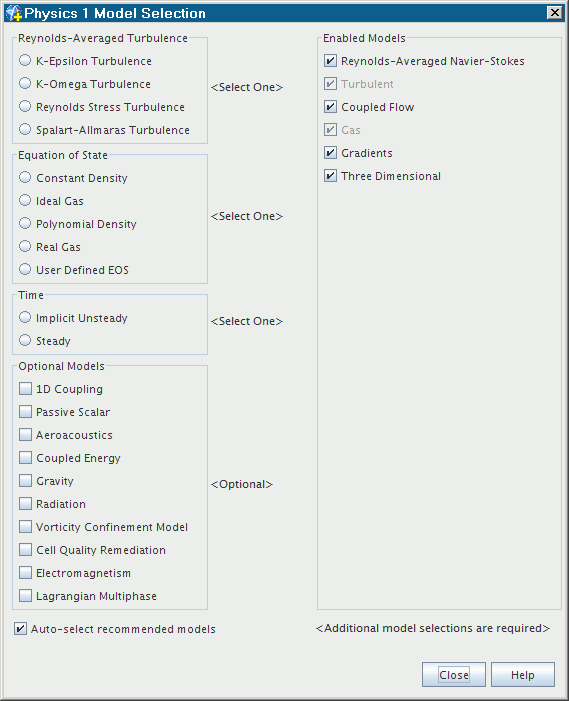
While the end result will depend on the choice made above, a complete selection will be made, including the variant of the model and the wall treatment model. The result for the K-Epsilon model is shown below.

Without Using Auto-Select

This section describes how to select a turbulence model that supports DES without using the automatic selection of recommended models feature.

Use this selection method if large eddy simulation or detached eddy simulation is desired. Note too that these two turbulence modeling approaches are only available if the following prerequisite models are selected:

the Three-Dimensional model;
the Implicit Unsteady model;
the Segregated Flow model.

To select the turbulence modeling approach, first select the viscous regime. In the Model Selection dialog, make sure that the Auto-select recommended physics models checkbox is cleared. Then, in the Turbulence group box, select the radio button corresponding to the appropriate turbulence method. The availability of methods depends partly on your selection of a time model.

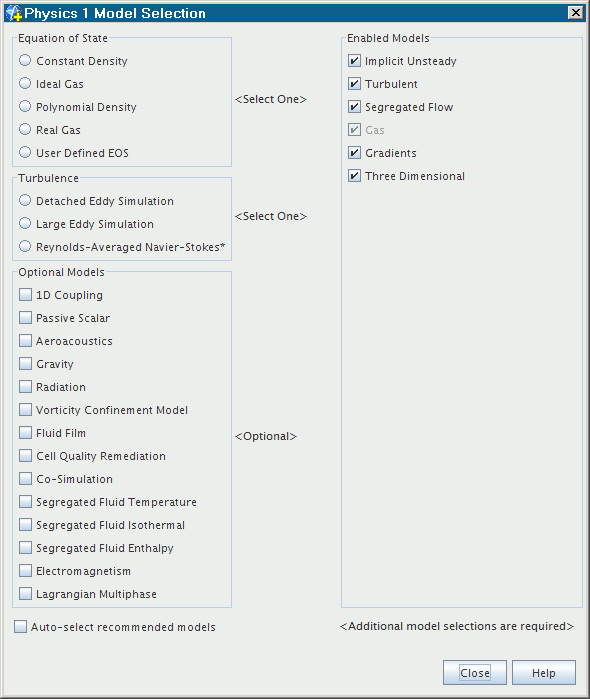
Depending on your selection, you will now be able to select:

a (RANS) turbulence model;
a subgrid scale model for large eddy simulation (LES); or
a turbulence model for detached eddy simulation.

The result for the SST K-Omega model is shown below.