Acoustic Modal Analysis

Simcenter STAR-CCM+ provides the Acoustic Modal Analysis model which allows you to simulate acoustic mode shapes, frequencies, and linear growth rates.

Acoustic pressure waves are generated in unsteady flows—for example, at turbulent wall boundaries, in shear layers, and in areas of vortex shedding. These acoustic pressure waves are of varying amplitude and frequency.

They propagate away from their sources within a geometry and can reflect off of boundaries back into the domain.

When acoustic pressure waves collide with a surface, the waves can reflect perfectly, or become damped and decrease in amplitude.

Within closed or partially closed domains, pressure waves can collide and their magnitudes be re-inforced—this leads to stationary pressure waves at the natural frequencies of the domain.

Typically, the lowest frequency standing waves are excited.

Such acoustic pressure waves may become unstable—for example, in combustion with unsteady heat release—and can cause damage to the structure.

The acoustic modes are the natural frequencies of the geometries and represent the standing (constant amplitude) acoustic waves.

The Acoustic Modal Solver in Simcenter STAR-CCM+ predicts these standing wave shapes, frequencies, and linear stability.

The following image shows examples of acoustic mode shapes that are simulated from a resonator box geometry in Simcenter STAR-CCM+. The scale shows the normalized acoustic pressure from 0.0 (blue) to 1.0 (red):

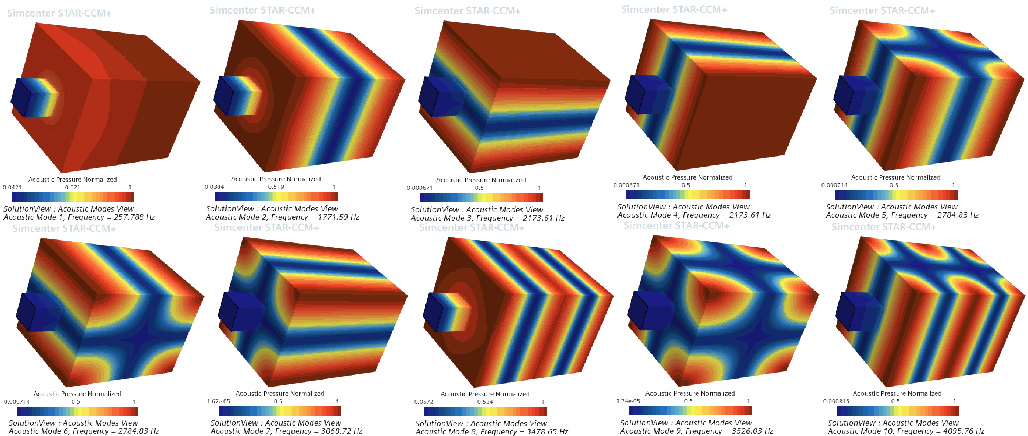
In addition to the mode shapes and frequencies, Simcenter STAR-CCM+ also predicts the linear growth rates (whether the waves are damped and stable, or amplified and unstable).

According to the Raleigh criterion, when heat is released in the geometry (for example, from combustion), and the unsteady heat release is in phase with the acoustic pressure waves, the acoustic pressure waves can also amplify—leading to thermo-acoustic instability.

The Acoustic Modal Analysis model in Simcenter STAR-CCM+ uses an n-tau model to model the effect of the unsteady heat release rate on the acoustic pressure. The n-tau model is a time delay model where the effect of the unsteady heat release on the local pressure is modeled as proportional to the heat release (by a factor n) delayed by some time (denoted tau). For example, a common mechanism of thermo-acoustic instability is unsteady heat release at a flame which generates acoustic waves that travel upstream to the fuel supply and cause a change in the fuel flow rate. This causes a change in the equivalence ratio, which is convected downstream to the flame zone and causes an oscillation in the heat release, repeating the cycle. The time delay in such a case is the convection time from the inlet to the flame.

Thermo-acoustic instabilities are present in most closed combustion devices and uncontrolled instabilities are detrimental to most devices. By identifying the operating conditions in which unstable modes originate, you can modify the geometry or change the operating conditions to prevent uncontrolled instabilities.